If you were given a choice to take £100 today or £100 a year from now, what would you decide to do? The answer should be ‘today.’ It seems an obvious answer, because why people should wait one year to get something they can have now. Nonetheless, the concept of the time value of money is an important principle in financial management. The value of money depends on when the cash flow occurs - £100 now is worth more than £100 at some future time (Pike et al., 2015).

In this article, we shall look at few concepts that are strictly associated with the theory of time value of money:
• Inflation;
• Simple Interest & Compound Interest;
• Present Value (PV) & Future Value (FV);
• Discounting & Net Present Value (NPV); and
• Opportunity Cost
Many reasons support the notion of money being worth more now than in the future:
Inflation. Gibson (2003) describes inflation as the systemic rise in goods and services' prices over time. In an economic view, inflation is caused by the relationship between the money supply and the economy's size. In other words, if the money supply (the amount
of money circulating in an economy) grows too fast relative to the size of the economy, the purchasing power of money falls, and prices rise. That is the reason why governments, more precisely national central banks, cannot excessively print new money. It would bring inflation to unsustainably high levels. The rise of prices can also be reflected in ''demand-pull'' inflation, where the increase in demand for a particular product or service exceeds an economy's production capacity. For instance, the high demand for residential properties in line with an insufficient number of houses available on the market will cause inflation.
So, going back to the question of whether you would prefer to receive £100 now or in a year, it leaves no doubt that under inflationary conditions, the value of money, in terms of its purchasing power over goods and services, would decline.
Hargreaves Lansdown website offers a calculator that shows the effect of inflation on the real value of your savings and the growth rate you would have needed to keep pace with inflation. For example, between January 2019 and today, the costs of goods and services increased roughly by 4.4%.
Figure 1. Hargreaves Lansdown: Inflation Calculator. Retrieved from https://www.hl.co.uk/tools/calculators/inflation-calculator

The calculator shows that for £100 needed to buy a certain basket of goods in January 2019, you would now have to spend £104 for the same products or services; thus, the purchasing power of money has decreased.
Time/ Investment. The second reason is that you can invest money you received today and earn interest, e.g., by putting money on your saving account or purchasing shares in a company. The type of investment a person chooses to make would generally depend on the level of risk they are willing to take.
If you do not want to invest money, you always have a possibility to spend it immediately or in the foreseeable future. The level of consumer spending is also an important factor contributing to the overall health of an economy.
Risk. Finally, if you have the money now, you definitely have money in your possession. The alternative of the promise of those £100 to be received in one year carries the risk that the payment may not be made at all or it will be less.
Simple Interest
Let us imagine you decided to take those £100 together with your total savings of £500 (£600 in total) and make an investment with a given annual rate of simple interest at 6%. Using the below formula, we can calculate what interest will be earned over a specified period of time on our principal amount of investment.
I=P(1+rt)P – the principal amount
r – interest rate
t – time
I=600(1+0.06x3)
I = 600 x 1.18 = £708
After three years, the generated interest derived from the investment would be £108, providing the full amount of £708. Nevertheless, simple interest is rarely used in real life. Almost all banks and other financial institutions use compound interest (Centre for Innovation in Mathematics Teaching, n.d.). According to the Corporate Finance Institute (CFI), simple interest is used in, e.g., mortgages where there is no compounding effect on the interest itself.
Compound Interest
Compound interest is interest earned on previously earned interest (HSBC, n.d). In other words, the process of compounding is the way to determine the future value of a sum of money invested now where additional interest is paid on the interest already received (Watson & Head, 2019). The formula used here is as follows:

FV – future value
Co – sum deposited now
i – annual interest rate
n – number of years for which the sum is invested
Figure 2. Compound interest explained. Available at https://www.hsbc.co.uk/savings/what-is-compound-interest/

In previous example, £600 invested received £108 of simple interest after three years of investment. How much more interest would have been made if instead of simple interest we were offered compound interest at the same annual rate (6%) per year?

Figure 3. Another explanation of compound interest (Pike, et.al, 2015)

Discounting
Discounting is the opposite of compounding. It is the act of estimating the present value of a future payment or a series of cash flows (Watson & Head, 2019). At times, it is necessary to find the present value of a sum of money available in the future. The calculations are made using the formula for calculating present value (PV).

PV – present value
FV – future value
i – discount rate
n – number of years until the cash flow occurs
Net Present Value
Net present value (NPV) is a popular method of discounted cash-flow (DCF) models and it is widely used in accounting and finance when assessing the feasibility of an investment, e.g. plants and equipment, financial securities or in real estate, and so on. This term is often referred as one of the investment appraisal or capital budgeting techniques.
‘‘Net present value is a method of a discounted cash-flow approach to capital budgeting that computes the present value of all expected future cash flows using a require rate of return.’’
Horngren, et al., (2017)
In simple words, NPV is the accumulation of all present values (single cash flows) of future discounted cash inflows and outflows (positive and negative cash flows) over a specified period of time. The formula for NPV is as the following:

The formula shows well how NPV consists of a series of the present value of future cash flows calculations. Let us imagine that we make an investment of £1000 and generate the following cash flows for the next five years. The graph below shows that the total amount of cash inflows is £1800 - generated in years 3-5 with a total of five years of investment. It looks like we made £800 of profit, thus making this project feasible and profitable. However, this assumption would be short-sighted as we have not considered the cost of capital. For this example, the proposed discount rate here is 8%.
Imagine that as a manager of a company you were asked to complete the investment appraisal using NPV method. Based solely on the results of your NPV analysis, would you be likely to go ahead with this investment?
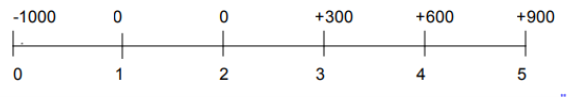

Based on the NPV analysis, which considered the costs of capital, inflation, etc., the five-year investment's net present value would be worth £1291.69, thus suggesting that the investment may be worth it as it generates profits (£291.69).
An alternative way to conduct NPV analysis is to use Excel spreadsheets. Corporate Finance Institute provides in his website a ready to go formula which looks like this (you can download it at https://corporatefinanceinstitute.com/resources/knowledge/valuation/net-present-value-npv/):
Figure 4. Corporate Finance Institute’s NPV formula

As we have already mentioned, the key concept of NPV analysis is a discount rate (otherwise known as hurdle rate or the required rate of return) that reflects three main factors: time, inflation, and risk. It would not make sense to add future cash inflows to the capital amount as they are subject to, e.g., inflation. Thus, the discount rate must be applied to future cash flows to obtain an objective present value. In simple terms, the NPV method basically suggests what investment would be worth today in real money if future cash flows were taken into consideration. The included required rate of return is the minimum acceptable rate of return calculated based on the firm's cost of capital. Her Majesty's Treasury guidance on appraising and evaluating policies, projects, and programs specifies a discount rate of 3.5% to be used for projects with a life span from 1 to 30 years (Green Book of HM Treasury. Available at https://assets.publishing.service.gov.uk/government/uploads/system/uploads/attachment_data/file/938046/The_Green_Book_2020.pdf). Of course, the required rate of return will differ for various entities. Cash inflows can also take the form of savings derived from an investment, e.g., if a new plant help to reduce its operational costs by £2000 a month, then effectively those £2000 is the cash-inflow.
Advantages of net present value
The NPV method considers the time value of money, which brings the notion that money today is worth more than money in the future. It is so because present money can be invested into an asset that can be sold for more, or money can be used to generate interest (an income) as in financial securities (bonds, shares, commodities, etc.). That is why this method can be advantageous in large projects, such as the purchase of expensive equipment or software and mergers & acquisitions where it is called discounted cash flow model (Harvard Business Review). It is a more sophisticated form of capital budgeting compared with accounting rate of return (ARR) or the payback period (PP), and it generally helps to see a 'bigger picture' when assessing investment.
DISADVANTAGES OF NET PRESENT VALUE
Some downsides or limitations of using NPV are that it is based on several assumptions, which creates a situation where mistakes can be made. For instance, there is a possibility that the interest rate will be higher in the future, resulting in the costs of funds rising as well, effectively making an investment less attractive than initially assessed.
The Common 'enemy' of all investments is the concept of an opportunity cost. In an economic context, the opportunity cost is discussed in line with the theory of scarcity (it means that resources are limited). Cash is a minimal resource, especially for many small and medium-sized companies. Therefore every investment, whether profitable or not, brings a question: 'Can those funds be spent on something else?' So, in essence, if you decide to make any investment, the opportunity cost 'guy' tells what you must give up for that choice.
Any Thoughts?
The time value of money is probably the single most important theory in finance (Watson & Head, 2019)… and is relevant for both corporations and individual investors. It is a fundamental concept that allows us to determine a stream of future cash flows whenever we conduct any financial investment. We may be able to understand if our bonds are likely to generate a desirable interest, assess whether our company should buy a piece of equipment or software or to place value on any financial security… and to do so, we must understand well the mathematics of time value of money (CFA Institute, n.d.)
To further understand the topic, you can refer to the podcast recorded by David Thorpe for the Financial Times: 'Inflation does not have to be bad for equities, where Phil Smeaton (Chief Investment Officer) at advice firm Sanlam discusses the implications of inflation on security prices: the outlook for inflation is a key focus for us. Asset prices are elevated, and valuations are high… The market's key focus is on whether interest rates rise, as that would likely mean asset prices fall, but rates will only rise if inflation goes above the 2 percent target, and there is no sign of that happening. The time value of money concept is present throughout this article.
All in all, the concept of the time value of money will appear again and again if you study any degree in the field of business, finance, or economics. Even if you are not a student and not interested in finance, a clear understanding of this concept may help us, to some extent- make better financial decisions. We would strongly recommend reading all resources in the 'Further reading' section as it will definitely help to understand the topic a lot better.
Further reading
Corporate Finance Institute (n.d.). Net Present Value (NPV) (online). Available at https://corporatefinanceinstitute.com/resources/knowledge/valuation/net-present-value-npv/
Gallo, A. (2014). Harvard Business Review. A Refresher on Net Present Value (online). Available at https://hbr.org/2014/11/a-refresher-on-net-present-value
Thorpe, D. (2020). FT Adviser. Inflation Rise Doesn’t Have to Be Bad For Equities’ (online). Available at https://www.ftadviser.com/investments/2021/01/22/inflation-rise-doesn-t-have-to-be-bad-for-equities/
Oner, C. (2010). International Monetary Fund. What is Inflation? (online). Available at https://www.imf.org/external/pubs/ft/fandd/2010/03/pdf/basics.pdf
Bibliography
Berk, J. and DeMarzo, P. (2019). Corporate Finance, Global Edit ion. 5th ed. [ebook] Pearson. Available at: https://www.perlego.com/book/971488/corporate-finance-global-edition-pdf
Pike, R., Neale, B. and Linsley, P. (2015). Corporate Finance and Investment: Decisions and Strategies. 8th ed. [ebook] Pearson. Available at: https://www.perlego.com/book/810751/corporate-finance-and-investment- decisions-and-strategies-pdf
Watson, D. and Head, A. (2019). Corporate Finance: Principles and Practice. 8th ed. [ebook] Pearson. Available at:https://www.perlego.com/book/811154/corporate-finance-principles-and-practice-pdf



Comments